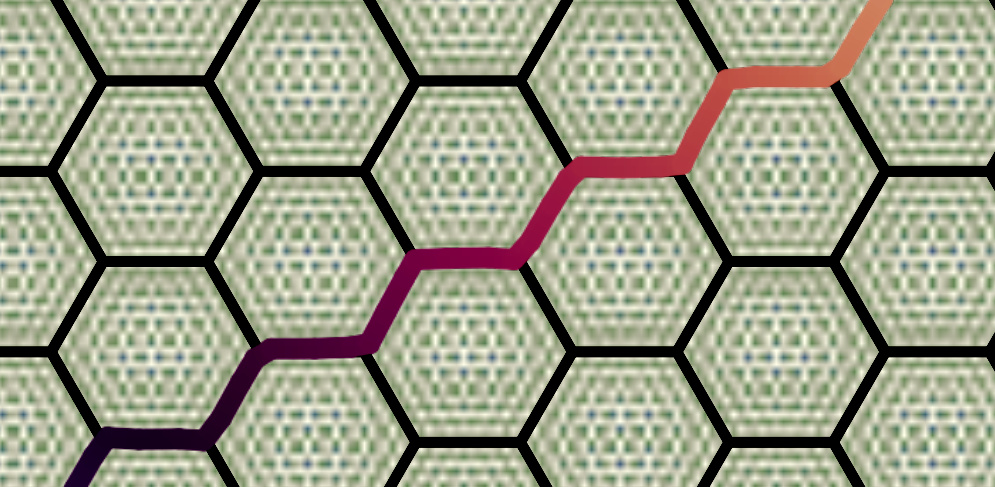
Competition between drift and topological transport of colloidal particles in twisted magnetic patterns
N. C. X. Stuhlmüller, T. M. Fischer, and D. de las Heras
New J. Phys., 26, 023056, (2024) DOI: 10.1088/1367-2630/ad2a81
Full text: journal, pdf
Abstract:
We simulate the motion of paramagnetic particles between two magnetic patterns with hexagonal symmetry that are twisted at a magic angle. The resulting Morié pattern develops flat channels in the magnetic potential along which colloidal particles can be transported via a drift force of magnitude larger than a critical value. Colloidal transport is also possible via modulation loops of a uniform external field with time varying orientation, in which case the transport is topologically protected. Drift and topological transport compete or cooperate giving rise to several transport modes. Cooperation makes it possible to move particles at drift forces weaker than the critical force. At supercritical drift forces the competition between the transport modes results e.g. in an increase of the average speed of the particles in integer steps and in the occurrence of subharmonic responses. We characterize the system with a dynamical phase diagram of the average particle speed as a function of the direction of the topological transport and the magnitude of the drift force.
Additional material/comments:
Download the full text for this article: journal, pdf
Related publications:
1 Enhanced colloidal transport in twisted magnetic patterns (+ info)
2 Colloidal transport in twisted lattices of optical tweezers (+ info)
3 Simultaneous and independent topological control of identical microparticles in non-periodic energy landscapes (+ info)
4 Magnetic colloidal single particles and dumbbells on a tilted washboard moiré pattern in a precessing external field (+ info)